Chapter 8: Linear inequalities

In Chapter 7 you learnt how to formulate and solve algebraic equations. An algebraic equation has an equals sign. We solve an equation when we find the variable that makes the equation true. We can represent an algebraic equation as a pair of scales, and the solution of the equation is the value that balances the scales.

In this chapter, you will learn how to solve linear inequalities. You can think of a linear inequality as a pair of scales that remains unbalanced.

8.1 Introduction to inequalities

Symbols that show relationships

We use the following symbols to show the relationship between two algebraic expressions:

$=$ means "equal to", for example $5-3=2$
$\neq$ means "not equal to", for example $10+1 \neq 5$
$\lt$ means "less than", for example $5 \lt 8+3$
$\gt$ means "greater than", for example $14-7\gt5$

To help you choose between the $\lt$ and $\gt$ symbols, remember that the symbol must "open up" towards the larger number. The "closed end" points to the smaller number. This crocodile may also help you remember which symbol to use. It always wants to eat the larger number, and turns away from the smaller number.

An algebraic inequality is a mathematical sentence that contains a combination of numbers, symbols, variables and mathematical operators. It always includes a "not equal to", "greater than" or "less than" symbol to show that two algebraic expressions are not equal to each other.

algebraic inequality An algebraic inequality is a mathematical sentence that includes a "not equal to", "greater than" or "less than" symbol to show that two algebraic expressions are not equal to each other.

Representing an inequality as a pair of scales

An algebraic inequality can be represented as a pair of scales that remains unbalanced. The scales below are unbalanced. There is nothing on the left-hand scale and there is a mass of 10 g on the right-hand scale.

Suppose we start putting mass pieces on the left-hand scale. If we want the left-hand scale to remain lighter than the right-hand scale, the mass on the left-hand scale must always be less than 10 g. We can write this as $m \lt 10\text{ g}$ .

If the mass on the left-hand scale reaches 10 g, the scales will balance, and we will no longer have an inequality.

Look at the pair of unbalanced scales below.

If we want the left-hand scale to remain lighter than the right-hand scale, $x + 1$ must always be smaller than 14. We can write this as $x + 1 \lt 14$ . It is easy to see that if $x = 13$ , the scales will be balanced. To keep the left-hand scale lighter, $x$ must be smaller than 13. We write this as $x \lt 13$ .

Values that make an inequality true

The algebraic equations you have dealt with so far have only one or two solutions. For example, the equation $x + 1 = 14$ has only one solution: $x = 13$ .

An algebraic inequality has more than one possible solution. For example, the inequality $x + 1 \lt 14$ has the solution $x \lt 13$ . This means any number smaller than 14 will make the inequality true. You can test this:

If $x = 10$ , then $10 + 1 \lt 14$ gives $11 \lt 14$ . This is true.
If $x = -2$ , then $-2 + 1 \lt 14$ gives $-1 \lt 14$ . This is true.
If $x = 13$ , then $13 + 1 \lt 14$ gives $14 \lt 14$ . This is false and shows that $x$ must be smaller than 13.
If $x = 16$ , then $16 + 1 \lt 14$ gives $17 \lt 14$ . This is false, because $x$ must be smaller than 13.

Worked example 8.1: Determining whether a solution makes an inequality true

If $x = 2$ , determine whether the inequality $3x - 5 \lt x + 4$ is true.

Step 1: Replace the variable in the inequality with the given value.
\begin{align} 3x-5& < x+4 \\ 3(2)-5& < 2+4 \end{align}
Step 2: Simplify both sides of the inequality.
\begin{align} 3x-5& < x+4 \\ 3(2)-5& < 2+4 \\ 6-5& < 6 \\ 1& < 6 \end{align}
Step 3: Evaluate the result of Step 2.

The number 1 is smaller than the number 6, so $x = 2$ is one of the values that makes the inequality true.

Exercise 8.1: Evaluate algebraic inequalities

Write the following word sentence as an algebraic inequality: "A length of 16 units is smaller than $y$ ."

16y>16
Write the following algebraic inequality in words: $a \gt 10$

The variable $a$ is greater than the number ten.
Put one of the symbols $\lt$ or $\gt$ in the box to make the inequality true: $7\times3 \; \square \; 28-2$

Insert the symbol < :
\begin{align} 7\times3&<28-2 \\ 21&<26 \\ \end{align}
State whether the following inequality is true or false: $-15+3\gt-2\times3+2$

\begin{align} -15+3&>-2\times3+2 \\ -12&>-6+2 \\ -12&>-4 \end{align}
Therefore, the inequality is false.

Remember that you must always follow the correct order of operations. Use BODMAS:Brackets, Of, Division, Multiplication, Addition, Subtraction.
State whether the following inequality is true or false for $x=-1$ : $10x+1\lt9+x+1$

\begin{align} 10x+1&<9+x+1 \\ 10(-1)+1&<9+(-1)+1 \\ -9&<9 \end{align}
Therefore, the inequality is true for $x=-1$ .

More symbols used in inequalities

The following symbols are also used to show relationships between two algebraic expressions:

$\leq$ means "less than or equal to"
$\geq$ means "greater than or equal to".

Look at the following pair of scales again.

If we represent this pair of scales with the inequality $x + 1 \leq 14$ , it means that the left-hand scale must be lighter than or in balance with the right-hand scale. We can therefore include the value that will balance the scales. This gives us a combination of two solutions:

the solution of the equation $x + 1 = 14$ , which is $x = 13$
the solution of the inequality x + 1 < 14 , which is x < 13

The combination is written as $x \leq 13$ .

8.2 Solving inequalities

The skills you used in Chapter 7 to solve equations can be used to solve inequalities.

If necessary, go back to Chapter 6 and revise how to expand brackets.

Worked example 8.2: Solving an inequality and checking the solution

Solve the following inequality and check the solution.
$-6(x-6)+4 \leq -8x-10$

Step 1: First expand brackets using the distributive law. If there are like terms on either side of the equation, collect them.
\begin{align} -6(x-6)+4 &\leq -8x-10 \\ -6x+36+4& \leq -8x-10 \\ -6x+40&\leq-8x-10 \end{align}
Step 2: Use additive inverses to arrange the equation so that all the terms with variables are on the left-hand side and all the constant terms are on the right-hand side.

Remember to do exactly the same on both sides of the equation.
\begin{align} -6(x-6)+4 &\leq -8x-10 \\ -6x+36+4& \leq -8x-10 \\ -6x+40&\leq-8x-10 \\ -6x+40{\color{red}{\;-\;40}}{\color{green}{\;+\;8x}} & \leq -8x{\color{green}{\;+\;8x}}-10 {\color{red}{\;-\;40}} \\ \end{align}
Step 3: Simplify both sides of the equation.
\begin{align} -6(x-6)+4 &\leq -8x-10 \\ -6x+36+4& \leq -8x-10 \\ -6x+40&\leq-8x-10 \\ -6x+40{\color{red}{\;-\;40}}{\color{green}{\;+\;8x}} & \leq -8x{\color{green}{\;+\;8x}}-10 {\color{red}{\;-\;40}} \\ -6x+8x &\leq -10-40 \\ 2x &\leq -50 \end{align}
Step 4: If the variable has a coefficient, apply the multiplicative inverse of the coefficient. Remember to do this on both sides of the equation.
\begin{align} -6(x-6)+4 &\leq -8x-10 \\ -6x+36+4& \leq -8x-10 \\ -6x+40&\leq-8x-10 \\ -6x+40{\color{red}{\;-\;40}}{\color{green}{\;+\;8x}} & \leq -8x{\color{green}{\;+\;8x}}-10 {\color{red}{\;-\;40}} \\ -6x+8x &\leq -10-40 \\ 2x &\leq -50 \\ {\color{orange}{\frac{1}{2}\times}} \frac{2x}{1}&\leq {\color{orange}{\frac{1}{2}\times}} \frac{-50}{1} \\ \end{align}
Step 5: Simplify both sides of the equation.
\begin{align} -6(x-6)+4 &\leq -8x-10 \\ -6x+36+4& \leq -8x-10 \\ -6x+40&\leq-8x-10 \\ -6x+40{\color{red}{\;-\;40}}{\color{green}{\;+\;8x}} & \leq -8x{\color{green}{\;+\;8x}}-10 {\color{red}{\;-\;40}} \\ -6x+8x &\leq -10-40 \\ 2x &\leq -50 \\ {\color{orange}{\frac{1}{2}\times}} \frac{2x}{1}&\leq{\color{orange}{\frac{1}{2}\times}}\frac{-50}{1} \\ \frac{2x}{2}&\leq \frac{-50}{2} \\ \therefore x&\leq-25 \end{align}
Step 6: Check the solution you found in Step 5. To check, write the original inequality as an equation, and substitute variables with the solution from Step 5.
\begin{align} -6(x-6)+4 &= -8x-10 \\ -6(-25-6)+4 &= -8(-25)-10 \\ -6(-31)+4&= -8(-25)-10 \\ 186+4 &= 200-10 \\ 190 &= 190 \\ \therefore \text{LHS}&=\text{RHS} \end{align}
The solution obtained in Step 5 is correct.
Step 7: Evaluate the solution. Test a number that adheres to the solution from Step 5. Replace the variables in the original inequality with the test number.

$x \leq -25$ , so we must use a number smaller than $-25$ . Let us use $-30$ .
\begin{align} -6(x-6)+4 &\leq -8x-10 \\ -6(-30-6)+4 &\leq -8(-30)-10 \\ -6(-36)+4&\leq -8(-30)-10 \\ 216+4 &\leq 240-10 \\ 220 &\leq 230 \\ \end{align}
This is true, so the solution is correct.

Using a multiplicative inverse that is negative

Look at the equation $-2x=6$ . To isolate the variable, we must use the multiplicative inverse of its coefficient:

\begin{align} {\color{red}{\frac{1}{-2}\times}} \frac{-2x}{1} & = \frac{6}{1}{\color{red}{\times\frac{1}{-2}}} \\ \frac{-2x}{-2}&=\frac{6}{-2} \\ x&=-3 \end{align}

If the above equation is changed to the inequality -2x<6 , we cannot simply replace the equals sign in the solution with a less than sign. If -2x<6 , then $x \nless-3$ . Test a number smaller than $-3$ , for example $-5$ :

\begin{align} -2x&<6 \\ -2(-5)&<6 \\ 10&<6 \end{align}

This solution is not true.

For algebraic inequalities, if we apply a negative multiplicative inverse or divide every term of the inequality by a negative number, the relationship symbol swaps around. For example:

$-x\gt1$ means that $x\lt-1$

$-x\lt-5$ means that $x\gt5$

Therefore:

\begin{align}\require{enclose} -2x&<6 \\ {\color{red}{\frac{1}{-2}\times}} \frac{-2x}{1} & {\color{red}{\enclose{circle}{>}}} \frac{6}{1}{\color{red}{\times\frac{1}{-2}}} \\ \frac{-2x}{-2}&>\frac{6}{-2} \\ x&>-3 \end{align}

Test a number larger than than $-3$ , for example $-1$ :

\begin{align} -2x&<6 \\ -2(-1)&<6 \\ 2&<6 \end{align}

This solution is true.

Worked example 8.3: Using a negative multiplicative inverse to solve an inequality

Solve the following inequality and check the solution.
$3x+5 \geq x-3+4x$

Step 1: Expand brackets and collect like terms.
\begin{align} 3x+5 &\geq x-3+4x \\ 3x+5 &\geq 5x-3 \\ \end{align}
Step 2: Use additive inverses to arrange the equation so that all the terms with variables are on the left-hand side and all the constant terms are on the right-hand side.
\begin{align} 3x+5 &\geq x-3+4x \\ 3x+5 &\geq 5x-3 \\ 3x+5{\color{red}{\;-\;5}}{\color{green}{\;-\;5x}}&\geq 5x{\color{green}{\;-\;5x}}-3 {\color{red}{\;-\;5}} \\ \end{align}
Step 3: Simplify both sides of the equation.
\begin{align} 3x+5 &\geq x-3+4x \\ 3x+5 &\geq 5x-3 \\ 3x+5{\color{red}{\;-\;5}}{\color{green}{\;-\;5x}}&\geq 5x{\color{green}{\;-\;5x}}-3 {\color{red}{\;-\;5}} \\ 3x-5x &\geq -8 \\ -2x &\geq -8 \end{align}
Step 4: If the variable has a coefficient, apply the multiplicative inverse of the coefficient. If the multiplicative inverse is negative, swap the relationship symbol around.
\begin{align} 3x+5 &\geq x-3+4x \\ 3x+5 &\geq 5x-3 \\ 3x+5{\color{red}{\;-\;5}}{\color{green}{\;-\;5x}}&\geq 5x{\color{green}{\;-\;5x}}-3 {\color{red}{\;-\;5}} \\ 3x-5x &\geq -8 \\ -2x &\geq -8 \\ {\color{orange}{\frac{1}{-2}\times}}\frac{-2x}{1}&{\color{red}{\enclose{circle}{\leq}}}{\color{orange}{\frac{1}{-2}\times}}\frac{-8}{1} \\ \end{align}
Step 5: Simplify both sides of the equation.
\begin{align} 3x+5 &\geq x-3+4x \\ 3x+5 &\geq 5x-3 \\ 3x+5{\color{red}{\;-\;5}}{\color{green}{\;-\;5x}}&\geq 5x{\color{green}{\;-\;5x}}-3 {\color{red}{\;-\;5}} \\ 3x-5x &\geq -8 \\ -2x &\geq -8 \\ {\color{orange}{\frac{1}{-2}\times}}\frac{-2x}{1}&{\color{red}{\enclose{circle}{\leq}}}{\color{orange}{\frac{1}{-2}\times}}\frac{-8}{1} \\ \frac{-2x}{-2}&\leq \frac{-8}{-2} \\ x&\leq 4 \end{align}
Step 6: Check the solution you obtained in Step 5. Write the original inequality as an equation, and substitute the solution for the variables.
\begin{align} 3x+5 &= x-3+4x \\ 3(4)+5 &= 4-3+4(4) \\ 12+5&=1+16 \\ 17 &= 17 \\ \therefore \text{LHS}&=\text{RHS} \end{align}
The solution obtained in Step 5 is correct.
Step 7: Evaluate the solution. Test a number that adheres to the solution from Step 5. Replace the variables in the original inequality with the test number.

$x\leq 4$ , so we must use a number smaller than 4. Let us use 3.
\begin{align} 3x+5 &\geq x-3+4x \\ 3(3)+5 &\geq 3-3+4(3) \\ 9+5&\geq 0+12 \\ 14 &\geq 12 \\ \end{align}
This is true. The solution is correct.

Exercise 8.2: Solve inequalities

Solve each of the following inequalities.

$4x \geq 14$
\begin{align} 4x &\geq 14 \\ \frac{4x}{4}&\geq \frac{14}{4} \\ x&\geq\frac{7}{2} \end{align}
$9\lt-3x$
\begin{align} 9&<-3x \\ \frac{9}{-3}&>\frac{-3x}{-3} \\ -3&>x \\ x&<-3 \\ \end{align}
$-3\gtx$ and x<-3 means exactly the same. If $-3$ is greater than $x$ , then $x$ must be less than $-3$ .
$x+5\gt10$
\begin{align} x+5&>10 \\ x+5-5&>10-5 \\ x&>5 \end{align}
$9x-6 \leq 21$
\begin{align} 9x-6 &\leq 21 \\ 9x-6+6 &\leq 21+6 \\ 9x&\leq 27\\ \frac{9x}{9} &\leq \frac{27}{9} \\ x&\leq 3 \end{align}
$4x+4 \gt 29-1+x$
\begin{align} 4x+4 &> 29-1+x \\ 4x+4-4-x &> 28+x-4-x \\ 3x &> 24 \\ \frac{3x}{3} &> \frac{24}{3} \\ x&>8 \end{align}
$5-2x \leq -17$
\begin{align} 5-2x &\leq -17 \\ 5-5-2x &\leq -17-5 \\ -2x &\leq -22 \\ \frac{-2x}{-2} &\geq \frac{-22}{-2} \\ x&\geq 11 \end{align}
$2x+1 \geq 3x+5$
\begin{align} 2x+1 &\geq 3x+5 \\ 2x+1-1-3x &\geq 3x-3x+5-1 \\ -x &\geq 4 \\ \frac{-x}{-1} &\leq \frac{4}{-1} \\ x&\leq -4 \end{align}
$2(5x+4)\gt14$
\begin{align} 2(5x+4)&>14 \\ 10x+8 &>14 \\ 10x+8-8 &> 14-8 \\ 10x&>6 \\ \frac{10x}{10} &> \frac{6}{10} \\ x&> \frac{3}{5} \end{align}
$4(x-9)+2 \gt 3(x+1)$
\begin{align} 4(x-9)+2 &> 3(x+1)\\ 4x-36+2&>3x+3 \\ 4x-34&>3x+3 \\ 4x-34+34-3x&>3x-3x+3+34 \\ x&>37 \\ \end{align}
$-2(x-2)+4\leq 18x+14$
\begin{align} -2(x-2)+4&\leq 18x+14 \\ -2x+4+4&\leq 18x+14 \\ -2x+8-8-18x&\leq 18x-18x+14-8 \\ -20x&\leq 6 \\ \frac{-20x}{-20} &\geq \frac{6}{-20} \\ x&\geq -\frac{3}{10} \end{align}

8.3 Showing inequalities on a number line

Last year you learnt that we can use a number line to represent integers. This is a straight line, divided into equal segments, that shows the position of numbers. We draw a number line with arrowheads on both ends to show that the line can carry on in both directions forever. Here is an example of a number line.

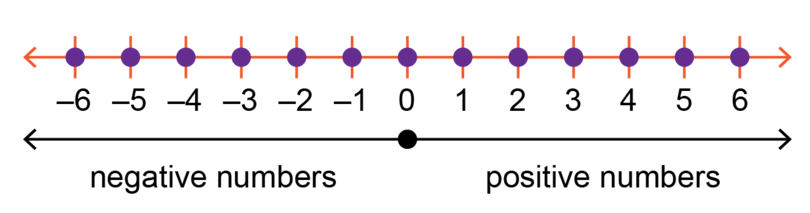
number line A number line is a straight line, divided into equal segments, that shows the relative position of numbers.

We can represent algebraic inequalities on a number line. It makes it easier to understand the solution of an inequality. When you use a number line to represent an inequality, follow these rules.

We use an arrow to show which numbers are included in the inequality:
- For numbers greater than a certain value, the arrow points to the right.
- For numbers less than a certain value, the arrow points to the left.
We use a circle to show where the arrow must start:
- An open circle means the number where the arrow starts is not included.
- A closed circle means the number where the arrow starts is included.

Here are some examples.

$x\gt2$

\[x\lt2\]

$x\leq -1$

$x\geq -1$

Worked example 8.4: Showing the solution of an inequality on a number line

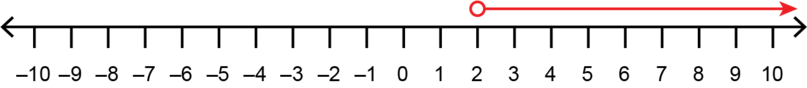
Solve the following inequality and show the solution on a number line.
$-1-4x\gt x+14$

Step 1: Solve the inequality.
\begin{align} -1-4x& \lt x+14 \\ -1+1-4x-x& \lt x+14-x+1 \\ -4x-x& \gt 14+1 \\ -5x& \gt 15 \\ \frac{-5x}{-5}& \lt \frac{15}{-5} \\ x& \lt -3 \end{align}
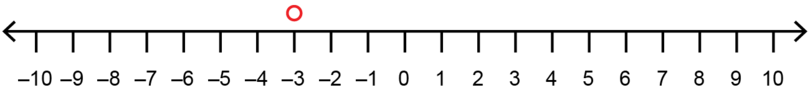
Step 2: Decide whether the circle where the arrow starts must be open or closed. Show it on a number line.

x<-3 means $-3$ is not included in the solution. The circle must be open.
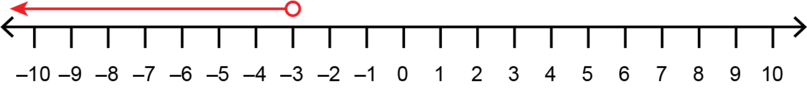
Step 3: Decide whether the arrow must point to the left or to the right.

x<-3 means $x$ is all the numbers smaller than $-3$ . The arrow must point to the left.

Exercise 8.3: Show the solutions of inequalities on a number line

Solve each of the following inequalities and show the solution on a number line.

$2\gt x-3$
\begin{align} 2&>x-3 \\ 2+3&>x-3+3 \\ 5&>x \\ x&<5 \end{align}
$4x-1\geq 11$
\begin{align} 4x-1&\geq 11 \\ 4x-1+1&\geq 11+1 \\ 4x&>12 \\ \frac{4x}{4}&\geq \frac{12}{4} \\ x&\geq3 \end{align}
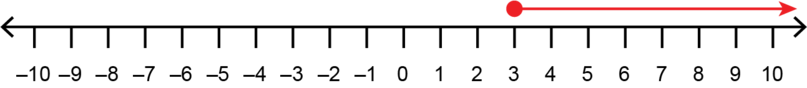
\[-7x\lt42\]
\begin{align} -7x&<42 \\ \frac{-7x}{-7}&> \frac{42}{-7} \\ x&>-6 \end{align}
\[5(x+1)\lt-15\]
\begin{align} 5(x+1)&<-15 \\ 5x+5&<-15 \\ 5x+5-5&<-15-5 \\ 5x&<-20 \\ \frac{5x}{5}&< \frac{-20}{5} \\ x&<-4 \end{align}
$4(x-2)\leq-2$
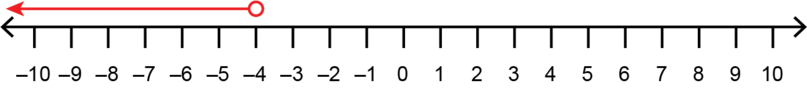
\begin{align} 4(x-2)&\leq-2 \\ 4x-8&\leq -2 \\ 4x-8+8& \leq -2+8 \\ 4x &\leq 6 \\ \frac{4x}{4} &\leq \frac{6}{4} \\ x&\leq\frac{3}{2} \\ x&\leq1\frac{1}{2} \end{align}

8.4 Practical applications

In Chapter 7 you learnt how you can use algebraic equations to solve problems. The same principles apply to algebraic inequalities.

If necessary, go back to Chapter 7 and revise how to use equations to solve problems.

Exercise 8.4: Use inequalities to solve problems

A student reads a book that has 288 pages. She reads $x$ pages every day. After 16 days, she has not finished reading the book. Determine the possible number of pages she read per day.

The student read $16x$ pages altogether, and she read less than the total of 288 pages.
\begin{align} 16x &< 288 \\ \frac{16x}{16} &< \frac{288}{16} \\ x &< 18 \end{align}
She read less than 18 pages per day.
A mother is twenty two years older than her son. In five years' time, she will still be younger than fifty years old. Determine the possible current age of the son.

Let the son's current age be $x$ . Then the mother's current age is $x+22$ .

In five years' time, the mother's age will be $x+22+5$ . This will be less than fifty.
\begin{align} x+22+5&<50 \\ x+27&<50 \\ x+27-27&<50-27 \\ x&<23 \\ \end{align}
The son is currently younger than 23 years.
If eight is subtracted from twice a certain number, the minimum answer is 30. Determine the possible values the number can have.

Let the number be $x$ .

If the minimum answer is 30, then $2x-8\geq30$ .
\begin{align} 2x-8&\geq30 \\ 2x-8+8&\geq30+8 \\ 2x&\geq38 \\ \frac{2x}{2}&\geq\frac{38}{2}\\ x&\geq19 \end{align}
If any number greater than or equal to 19 is doubled and then 8 is subtracted, the answer will be 30 or more.
A rectangular area on the schoolgrounds must be fenced in such a way that the breadth of the area is 50 metres less than the length of the area. The school has only 340 metres of fencing wire. Determine the maximum breadth that the area can have.

Let the breadth be $x$ .

Then the length is $x+50$ .

The total perimeter of the rectangle must be 340 or less.

Remember, the perimeter of a rectangle is given by $P=2l+2b$ .
\begin{align} 2(x+50)+2x&\leq 340 \\ 2x+100+2x&\leq 340 \\ 4x+100&\leq 340 \\ 4x+100-100&\leq 340-100 \\ 4x&\leq 240 \\ \frac{4x}{4} &\leq \frac{240}{4} \\ x &\leq 60 \end{align}
The breadth must be less than or equal to 60 m.
A certain number $x$ is a whole number. If a quarter of $x$ is subtracted from 2, the answer is always larger than 1. Determine the largest value that $x$ can have.

$2-\frac{1}{4}x$ must be larger than 1.
\begin{align} 2-\frac{1}{4}x&>1 \\ 2-2-\frac{1}{4}x&>1-2 \\ \frac-{1}{4}x&>-1 \\ \frac{-4}{1}\times\frac{-1}{4}x&<-1\times\frac{-4}{1} \\ x&<4 \\ \end{align}
If $x$ is a whole number, the largest value it can have is 3.

8.5 Summary

An algebraic inequality is a mathematical sentence that includes a "not equal to", "greater than" or "less than" symbol to show that two algebraic expressions are not equal to each other.
We use the following symbols in algebraic inequalities:
- $\neq$ means "not equal to"
- $\lt$ means "less than"
- $\gt$ means "greater than"
- $\leq$ means "less than or equal to"
- $\geq$ means "greater than or equal to".
If we apply a negative multiplicative inverse or divide every term of the inequality by a negative number, the relationship symbol swaps around. For example, $-x\gt1$ means that $x\lt-1$ .
We can represent algebraic inequalities on a number line.
On a number line, we use an arrow to show which numbers are included in the inequality:
- For numbers greater than a certain value, the arrow points to the right.
- For numbers less than a certain value, the arrow points to the left.
On a number line, we use a circle to show where the arrow must start:
- An open circle means the number where the arrow starts is not included.
- A closed circle means the number where the arrow starts is included.
We can use inequalities to solve problems.

Chapter 7: Simple equations

Table of Contents

Chapter 9: Graphs