Chapter 1: Numbers big and small

1.1 Standard form

Last year you learnt how the base ten number system, also called the decimal number system, works. In this system, we use the digits 0; 1; 2; 3; 4; 5; 6; 7; 8; 9 to represent any number, no matter how big or how small.

We may use powers of ten to express certain numbers. You dealt with the powers of ten listed in the table.
\begin{array}{|l|l|l|} \hline \textbf{Name} & \textbf{Number} & \textbf{Powers of ten} \newline \hline \text{Ten} & 10 & 10=10^1 \newline \hline \text{Hundred} & 100 & 10\times 10=10^2 \newline \hline \text{Thousand} & 1,000 & 10\times 10\times 10=10^3 \newline \hline \text{Ten thousand} & 10,000 & 10\times 10\times 10\times 10=10^4 \newline \hline \text{Hundred thousand} & 100,000 & 10\times 10\times 10\times 10\times 10=10^5 \newline \hline \text{Million} & 1,000,000 & 10\times 10\times 10\times 10\times 10\times 10=10^6 \newline \hline \text{Ten million} & 10,000,000 & 10\times 10\times 10\times 10\times 10\times 10\times 10=10^7 \newline \hline \text{Hundred million} & 100,000,000 & 10\times 10\times 10\times 10\times 10\times 10\times 10\times 10=10^8 \newline \hline \text{Billion} & 1,000,000,000 & 10\times 10\times 10\times 10\times 10\times 10\times 10\times 10\times 10=10^9 \newline \hline \text{Ten billion} & 10,000,000,000 & 10\times 10\times 10\times 10\times 10\times 10\times 10\times 10\times 10\times 10=10^{10} \newline \hline \text{Hundred billion} & 100,000,000,000 & 10\times 10\times 10\times 10\times 10\times 10\times 10\times 10\times 10\times 10\times 10=10^{11} \newline \hline \text{Trillion} & 1,000,000,000,000 & 10\times 10\times 10\times 10\times 10\times 10\times 10\times 10\times 10\times 10\times 10\times 10=10^{12} \newline \hline \end{array}

Fractions may also be written as powers of ten. You worked with this list last year:
\begin{array}{|l|l|l|} \hline \textbf{Name} & \textbf{Fraction} & \textbf{Powers of ten} \newline \hline \text{One tenth} & 0.1\;\text{or}\;\frac{1}{10} & \frac{1}{10}=10^{-1} \newline \hline \text{One hundredth} & 0.01\;\text{or}\;\frac{1}{100} & \frac{1}{10\times 10}=10^{-2} \newline \hline \text{One thousandth} & 0.001\;\text{or}\;\frac{1}{1,000} & \frac{1}{10\times 10\times 10}=10^{-3} \newline \hline \text{One ten thousandth} & 0.0001\;\text{or}\;\frac{1}{10,000} & \frac{1}{10\times 10\times 10\times 10}=10^{-4} \newline \hline \end{array}

Very large or very small numbers are often expressed in standard form. A number is in standard form when it is written as the product of any number between 1 and 10 (including 1) and a power of ten. Examples are $2.5\times 10^5$ and $8.2\times 10^{-2}$ .

Standard form is also called scientific notation.

The general format for standard from is: $a\times 10^n$ , where 1\leqslant a<10 and $n$ is an integer. Therefore, if we want to write a number in standard form, it must be written like this:

$\fbox{number 1 or larger, but smaller than 10}\space\times\space 10\,^\fbox{integer}$

standard form In standard form, a number is written in the form $a\times 10^n$ , where 1\leqslant a<10 and $n$ is an integer.

Integers include all the negative counting numbers, zero and all the positive counting numbers. It is the set $\lbrace \ldots -3; -2; -1; 0; 1; 2; 3 \ldots \rbrace$ .

Expressing whole numbers in standard form

It is generally easier to read and work with large numbers when they are written in standard form. Here are some examples:

The speed of light in space is 300,000,000 m/s. This can be written as $3\times 10^8 \text{m/s}$ .
In May 2019, the continent of Africa had a population of just over 1,300,000,000. This can be written as $1.3\times 10^9$ .
The amount of water in the Earth's oceans is estimated as 1,332,000,000,000. This can be written as $1.332\times 10^{12}$ .

When we express whole numbers in standard from, the power of ten is positive. Therefore, the format is:

$\fbox{number 1 or larger, but smaller than 10}\space\times\space 10\,^\fbox{positive integer}$

Worked example 1.1: Writing large numbers in standard form

Write the number 40,500,000 in standard form.

Step 1: Write down the general expression for whole numbers in standard form.
$\fbox{number 1 or larger, but smaller than 10}\space\times\space 10\,^\fbox{positive integer}$
Step 2: Write down the digits from the first non-zero digit to the last non-zero digit. Insert a decimal point between these digits. You must get a number that is equal to or larger than 1, but smaller than 10.

From the first to the last non-zero digit: $405$
Insert the decimal point: $4.05$
Now you have:
$4.05\times 10\,^\fbox{positive integer}$
Step 3: Work out how many positions the decimal point was moved to get from the original number to the number in Step 2.

The decimal point was moved 7 positions:
\begin{array}{c c c c c c c c} 4.& 0 & 5 & 0 & 0 & 0 & 0 & 0.\newline &\hookleftarrow &\hookleftarrow &\hookleftarrow &\hookleftarrow &\hookleftarrow &\hookleftarrow &\hookleftarrow \newline & ^7 & ^6 & ^5 & ^4 & ^3 & ^2 & ^1 \end{array}
Any whole number can be written with a decimal point right at the end. For example, the number 25 can be written as 25.0 and the number 110 can be written as 110.0.
Step 4: The power of ten is equal to the number of positions that the decimal point moved.

The decimal point moved 7 positions to the left. Therefore, standard form of the number 40,500,000 is:
$4.05\times 10^7$

To get from 40,500,000 to 4.05, we actually divided by $10^7$ :
$\require{cancel}\frac{40,500,000}{10\times 10\times 10\times 10\times 10\times 10\times 10}=\frac{40,5\!\cancel{00,000}}{10,0\!\cancel{00,000}}=\frac{405}{100}=4.05$
Therefore, to get to the original number again, we have to "multiply back" the $10^7$ .

Exercise 1.1: Convert between ordinary whole numbers and standard form

Write the numbers given in standard form as ordinary whole numbers.
1. $4\times 10^6$
$\begin{array}{l} 4\times 10\times 10\times 10\times 10\times 10\times 10 \newline =4,000,000 \end{array}$
1. $6.8\times 10^8$
$\begin{array}{l} 6.8\times 10\times 10\times 10\times 10\times 10\times 10\times 10\times 10 \newline =68\times 10\times 10\times 10\times 10\times 10\times 10\times 10\newline =680,000,000 \end{array}$
1. $2.22\times 10^7$
$\begin{array}{l} 2.22\times 10\times 10\times 10\times 10\times 10\times 10\times 10 \newline =222\times 10\times 10\times 10\times 10\times 10\newline =22,200,000 \end{array}$
1. $1.03\times 10^5$
$\begin{array}{l} 1.03\times 10\times 10\times 10\times 10\times 10 \newline =103\times 10\times 10\times 10\newline =103,000 \end{array}$
1. $3.007\times 10^9$
$\begin{array}{l} 3.007\times 10\times 10\times 10\times 10\times 10\times 10\times 10\times 10\times 10 \newline =3007\times 10\times 10\times 10\times 10\times 10\times 10\newline =3,007,000,000 \end{array}$
Write the whole numbers in standard form.
1. 20,000,000,000
$2\times 10\,^\fbox{positive integer}$
The decimal point moved 10 positions to the left.
Standard form is: $2\times 10^{10}$
1. 580,000,000
$5.8\times 10\,^\fbox{positive integer}$
The decimal point moved 8 positions to the left.
Standard form is: $5.8\times 10^8$
1. 33,330,000,000,000
$3.333\times 10\,^\fbox{positive integer}$
The decimal point moved 13 positions to the left.
Standard form is: $3.333\times 10^{13}$
1. 40,520,000,000
$4.052\times 10\,^\fbox{positive integer}$
The decimal point moved 10 positions to the left.
Standard form is: $4.052\times 10^{10}$
1. 720,720,000,000,000
$7.2072\times 10\,^\fbox{positive integer}$
The decimal point moved 14 positions to the left.
Standard form is: $7.2072\times 10^{14}$

Expressing decimal fractions in standard form

A decimal fraction is a fraction that is expressed using a decimal point. Instead of writing the fraction as one number on top of another other, $\frac{\text{numerator}}{\text{denominator}}$ , we write the numerator after a decimal point. We automatically know that the denominator is one of 10; 100; 1,000; 10,000 and so on. For example, the decimal fraction 0.2 means the numerator is 2 and the denominator is 10, so we may write it as $\frac{2}{10}$ .

Very small decimal fractions are often expressed in standard form. When we express decimal fractions in standard form, the power of ten is negative. Therefore, the format is:

$\fbox{number 1 or larger, but smaller than 10}\space\times\space 10\,^\fbox{negative integer}$

Worked example 1.2: Writing decimal fractions in standard form

Write the number 0,00000405 in standard form.

Step 1: Write down the general expression for decimal fractions in standard form.
$\fbox{number 1 or larger, but smaller than 10}\space\times\space 10\,^\fbox{negative integer}$
Step 2: Write down the digits from the first non-zero digit to the last non-zero digit. Insert a decimal point between these digits. You must get a number that is equal to or larger than 1, but smaller than 10.

From the first to the last non-zero digit: $405$
Insert the decimal point: $4.05$
Now you have:
$4.05\times 10\,^\fbox{negative integer}$
Step 3: Work out how many positions the decimal point was moved to get from the original number to the number in Step 2.

The decimal point was moved 6 positions:
\begin{array}{c c c c c c c c c} 0.& 0 & 0 & 0 & 0 & 0 & 4. & 0 & 5\newline &\hookrightarrow &\hookrightarrow &\hookrightarrow &\hookrightarrow &\hookrightarrow &\hookrightarrow & \newline & ^1 & ^2 & ^3 & ^4 & ^5 & ^6 & \end{array}
Step 4: The power of ten is equal to the number of positions that the decimal point moved.

The decimal point moved 6 positions to the right. Therefore, standard form of the number 0,00000405 is:
$4.05\times 10^{-6}$

To get from 0,00000405 to 4.05, we actually multiplied by $10^6$ :
$0,00000405\times 10\times 10\times 10\times 10\times 10\times 10=0,00000405\times 1,000,000=4.05$
Therefore, to get to the original number again, we have to "divide away" the $10^6$ .
Dividing by $10^6$ is the same as multiplying by $10^{-6}$ :
$\frac{4.05}{10^6}=4.05\times 10^{-6}$

Exercise 1.2: Convert between ordinary decimal fractions and standard form

Write the numbers given in standard form as ordinary decimal fractions.
1. $8\times 10^{-5}$
\begin{array}{l l} \frac{8}{10^5} &=\frac{8}{10\times 10\times 10\times 10\times 10} \newline &=0.00008 \end{array}
1. $2.4\times 10^{-4}$
\begin{array}{l l} \frac{2.4}{10^4} &=\frac{2.4}{10\times 10\times 10\times 10} \newline &=0.00024 \end{array}
1. $7.77\times 10^{-3}$
\begin{array}{l l} \frac{7.77}{10^3} &=\frac{7.77}{10\times 10\times 10} \newline &=0.00777 \end{array}
1. $9.05\times 10^{-5}$
\begin{array}{l l} \frac{9.05}{10^5} &=\frac{9.05}{10\times 10\times 10\times 10\times 10} \newline &=0.0000905 \end{array}
1. $1.004\times 10^{-3}$
\begin{array}{l l} \frac{1.004}{10^3} &=\frac{1.004}{10\times 10\times 10} \newline &=0.001004 \end{array}
Write the decimal fractions in standard form.
1. 0.0000006
$6\times 10\,^\fbox{negative integer}$
The decimal point moved 7 positions to the right.
Standard form is: $6\times 10^{-7}$
1. 0.000028
$2.8\times 10\,^\fbox{negative integer}$
The decimal point moved 5 positions to the right.
Standard form is: $2.8\times 10^{-5}$
1. 0.00000501
$5.01\times 10\,^\fbox{negative integer}$
The decimal point moved 6 positions to the right.
Standard form is: $5.01\times 10^{-6}$
1. 0.00009393
$9.393\times 10\,^\fbox{negative integer}$
The decimal point moved 5 positions to the right.
Standard form is: $9.393\times 10^{-5}$
1. 0.000005555
$5.555\times 10\,^\fbox{negative integer}$
The decimal point moved 6 positions to the right.
Standard form is: $5.555\times 10^{-6}$

1.2 Prime factorisation

Last year you learnt that a prime number has only two factors: 1 and the number itself. The prime numbers between 1 and 100 are highlighted in the grid below.

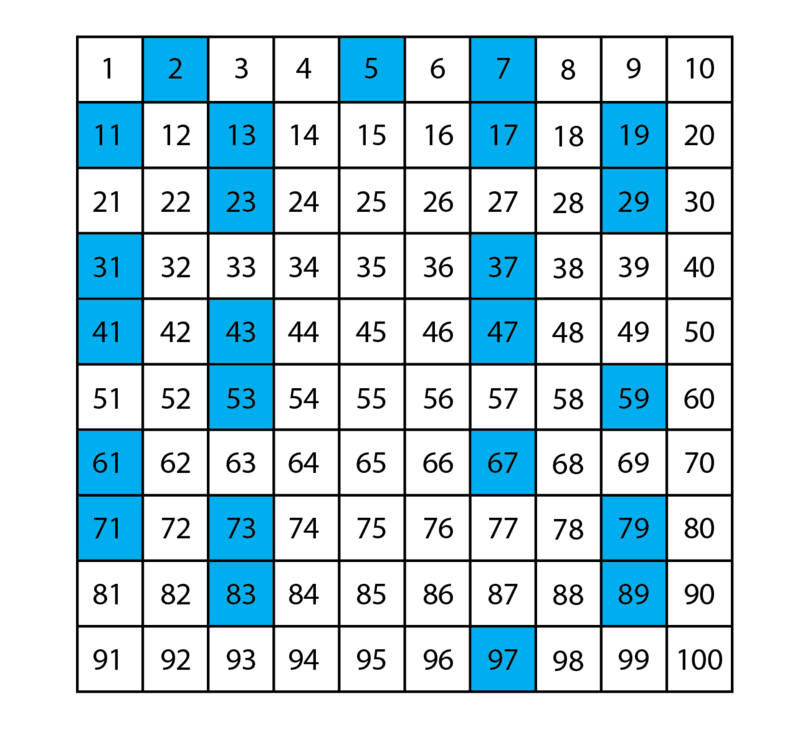
You also learnt that a prime factor is a prime number that divides into a non-prime number without a remainder. Any non-prime number can be expressed as a product of its prime factors.

prime factor A prime factor is prime number that divides into a non-prime number without a remainder.

We use index form to make it easier to express large non-prime numbers as products of their prime factors. The index or power of a number tells us how many times we multiply the number by itself.

index form In index form, we write how many times a number is multiplied by itself as a superscript next to the number, for example $3^2$ means $3\times 3$ .

Worked example 1.3: Revising prime factors

Express 180 as a product of its prime factors. Then write the prime factors in index form.

Method 1

Find the smallest prime factor of 180. Express 180 as the product of two factors.

Find the smallest prime factor of the non-prime factor from the previous step. Express 180 as the product of three factors.

Repeat the process until all the factors are prime numbers.

\begin{array}{r l} 180&=2\times 90\newline &=2\times 2\times 45\newline &=2\times 2\times 3\times 15\newline &=2\times 2\times 3\times 3\times 5\newline 180&=2^2\times 3^2\times 5 \end{array}

Method 2

Divide the smallest prime factor of 180 until it cannot divide further.

Move to the next prime factor and repeat.

Repeat the process until you get 1 as an answer.

\begin{array}{r | r} 2 & 180 \newline \hline 2 & 90 \newline \hline 3 & 45 \newline \hline 3 & 15 \newline \hline 5 & 5 \newline \hline & 1 \end{array} \begin{array}{r l} 180&=2\times 2\times 3\times 3\times 5\newline &=2^2\times 3^2\times 5 \end{array}

Exercise 1.3: Express numbers as products of prime factors in index form

Express the following numbers as prime factors in index form:

60

\begin{array}{r | r} 2 & 60 \newline \hline 2 & 30 \newline \hline 3 & 15 \newline \hline 5 & 5 \newline \hline & 1 \end{array} $60=2^2 \times 3 \times 5$
144

\begin{array}{r | r} 2 & 144 \newline \hline 2 & 72 \newline \hline 2 & 36 \newline \hline 2 & 18 \newline \hline 3 & 9 \newline \hline 3 & 3 \newline \hline & 1 \end{array} $144=2^4 \times 3^2$
198

\begin{array}{r | r} 2 & 198 \newline \hline 3 & 99 \newline \hline 3 & 33 \newline \hline 11 & 11 \newline \hline & 1 \end{array} $198=2\times 3^2 \times 11$
300

\begin{array}{r | r} 2 & 300 \newline \hline 2 & 150 \newline \hline 3 & 75 \newline \hline 5 & 25 \newline \hline 5 & 5 \newline \hline & 1 \end{array} $300=2^2 \times 3\times 5^2$
3,375

\begin{array}{r | r} 3 & 3,375 \newline \hline 3 & 1,125 \newline \hline 3 & 375 \newline \hline 5 & 125 \newline \hline 5 & 25 \newline \hline 5 & 5 \newline \hline & 1 \end{array} $3,375=3^3 \times 5^3$

1.3 Applications of prime factorisation

Lowest common multiple (LCM)

You have learnt that the lowest common multiple (LCM) of two or more numbers is the smallest number that is a multiple of all the numbers.

lowest common multiple (LCM) The lowest common multiple is the smallest whole number into which two or more numbers divide without a remainder.

Worked example 1.4: Revising LCM

Determine the LCM of 48, 72 and 120.

For both methods, we must find the prime factors of each number.
\begin{array}{r | r} 2 & 48 \newline \hline 2 & 24 \newline \hline 2 & 12 \newline \hline 2 & 6 \newline \hline 3 & 3 \newline \hline & 1 \end{array} $48=2\times 2\times 2\times 2\times 3=2^4\times 3$ \begin{array}{r | r} 2 & 72 \newline \hline 2 & 36 \newline \hline 2 & 18 \newline \hline 3 & 9 \newline \hline 3 & 3 \newline \hline & 1 \end{array} $72=2\times 2\times 2\times 3\times 3=2^3\times 3^2$ \begin{array}{r | r} 2 & 120 \newline \hline 2 & 60 \newline \hline 2 & 30 \newline \hline 3 & 15 \newline \hline 5 & 5 \newline \hline & 1 \end{array} $120=2\times 2\times 2\times 3\times 5=2^3\times 3\times 5$
Method 1

Express each number as a product of its prime factors.

Identify the smallest prime factors that must be included in a common multiple.

Multiply the prime factors from the previous step.

$48=2\times 2\times 2\times 2\times 3$
$72=2\times 2\times 2\times 3\times 3$
$120=2\times 2\times 2\times 3\times 5$

Any multiple of 48 must contain $2\times 2\times 2\times 2$ ( $3$ is included in $3\times 3$ below)
Any multiple of 72 must contain $3\times 3$ ( $2\times 2\times 2$ is included in $2\times 2\times 2\times 2$ above)
Any multiple of 120 must contain $5$ ( $3$ is included in $3\times 3$ above; $2\times 2\times 2$ is included in $2\times 2\times 2\times 2$ above)
$\therefore \text{LCM}=2\times 2\times 2\times 2\times 3\times 3\times 5=720$

Method 2

Express each number as a product of its prime factors, written in index form.

List all the prime factors that appear, to their highest index.

Multiply the prime factors from the previous step.

$48=2^4\times 3$
$72=2^3\times 3^2$
$120=2^3\times 3\times 5$

Highest index of the prime factor 2: $2^4$
Highest index of the prime factor 3: $3^2$
Highest index of the prime factor 5: $5$
$\therefore \text{LCM}=2^4\times 3^2\times 5=2\times 2\times 2\times 2\times 3\times 3\times 5=720$

Exercise 1.4: Determine LCM

Determine the LCM of the following sets of numbers.

24 and 60

\begin{array}{r | r} 2 & 24 \newline \hline 2 & 12 \newline \hline 2 & 6 \newline \hline 3 & 3 \newline \hline & 1 \end{array} $24=2^3 \times 3$ \begin{array}{r | r} 2 & 60 \newline \hline 2 & 30 \newline \hline 3 & 15 \newline \hline 5 & 5 \newline \hline & 1 \end{array} $60=2^2 \times 3 \times 5$ $\therefore \text{LCM}=2^3\times 3\times 5=2\times 2\times 2\times 3\times 5=120$
12, 18 and 40

\begin{array}{r | r} 2 & 12 \newline \hline 2 & 6 \newline \hline 3 & 3 \newline \hline & 1 \end{array} $12=2^2 \times 3$ \begin{array}{r | r} 2 & 18 \newline \hline 3 & 9 \newline \hline 3 & 3 \newline \hline \end{array} $18=2\times 3^2$ \begin{array}{r | r} 2 & 40 \newline \hline 2 & 20 \newline \hline 2 & 10 \newline \hline 5 & 5 \newline \hline & 1 \end{array} $40=2^3 \times 5$ $\therefore \text{LCM}=2^3\times 3^2\times 5=2\times 2\times 2\times 3\times 3\times 5=360$
45, 60 and 75

\begin{array}{r | r} 3 & 45 \newline \hline 3 & 15 \newline \hline 5 & 5 \newline \hline & 1 \end{array} $45=3^2 \times 5$ \begin{array}{r | r} 2 & 60 \newline \hline 2 & 30 \newline \hline 3 & 15 \newline \hline 5 & 5 \newline \hline & 1 \end{array} $60=2^2 \times 3 \times 5$ \begin{array}{r | r} 3 & 75 \newline \hline 5 & 25 \newline \hline 5 & 5 \newline \hline & 1 \end{array} $75=3 \times 5^2$ $\therefore \text{LCM}=2^2\times 3^2\times 5^2=2\times 2\times 3\times 3\times 5\times 5=900$

Highest common factor (HCF)

You have learnt that the highest common factor (HCF) of two or more numbers is the largest number that is a factor of all the numbers.

highest common factor (HCF) The highest common factor is the largest whole number that divides into two or more numbers without a remainder.

Worked example 1.5: Revising HCF

Determine the HCF of 24, 36, and 56

For both methods, we must find the prime factors of each number.
\begin{array}{r | r} 2 & 24 \newline \hline 2 & 12 \newline \hline 2 & 6 \newline \hline 3 & 3 \newline \hline & 1 \end{array} $24=2\times 2\times 2\times 3=2^3\times 3$ \begin{array}{r | r} 2 & 36 \newline \hline 2 & 18 \newline \hline 3 & 9 \newline \hline 3 & 3 \newline \hline & 1 \end{array} $36=2\times 2\times 3\times 3=2^2\times 3^2$ \begin{array}{r | r} 2 & 56 \newline \hline 2 & 28 \newline \hline 2 & 14 \newline \hline 7 & 7 \newline \hline & 1 \end{array} $56=2\times 2\times 2\times 7=2^3\times 7$
Method 1

Express each number as a product of its prime factors.

Identify the prime factors that are contained in all the numbers.

Multiply the prime factors from the previous step.

$24=2\times 2\times 2\times 3$
$36=2\times 2\times 3\times 3$
$56=2\times 2\times 2\times 7$

The number 2 appears twice in all the numbers: $2\times 2$ .
The number 3 does not appear for 56.
The number 7 does not appear for 24 or 36.
$\therefore \text{HCF}=2\times 2=4$

Method 2

Express each number as a product of its prime factors, written in index form.

List only the prime factors that are contained in all the numbers, to their lowest index.

Multiply the prime factors from the previous step.

$24=2^3\times 3$
$36=2^2\times 3^2$
$56=2^3\times 7$

The only prime factor that appears for all the numbers is 2. Its lowest index is 2.
$\therefore \text{HCF}=2^2=2\times 2=4$

Exercise 1.5: Determine HCF

Determine the HCF of the following sets of numbers.

40 and 64

\begin{array}{r | r} 2 & 40 \newline \hline 2 & 20 \newline \hline 2 & 10 \newline \hline 5 & 5 \newline \hline & 1 \end{array} $40=2^3 \times 5$ \begin{array}{r | r} 2 & 64 \newline \hline 2 & 32 \newline \hline 2 & 16 \newline \hline 2 & 8 \newline \hline 2 & 4 \newline \hline 2 & 2 \newline \hline & 1 \end{array} $64=2^6$ $\therefore \text{HCF}=2^3=2\times 2\times 2=8$
30, 45 and 75

\begin{array}{r | r} 2 & 30 \newline \hline 3 & 15 \newline \hline 5 & 5 \newline \hline & 1 \end{array} $30=2\times 3 \times 5$ \begin{array}{r | r} 3 & 45 \newline \hline 3 & 15 \newline \hline 5 & 5 \newline \hline & 1 \end{array} $45=3^2 \times 5$ \begin{array}{r | r} 3 & 75 \newline \hline 5 & 25 \newline \hline 5 & 5 \newline \hline & 1 \end{array} $75=3 \times 5^2$ $\therefore \text{HCF}=3\times 5=15$
96, 160 and 224

\begin{array}{r | r} 2 & 96 \newline \hline 2 & 48 \newline \hline 2 & 24 \newline \hline 2 & 12 \newline \hline 2 & 6 \newline \hline 3 & 3 \newline \hline & 1 \end{array} $96=2^5\times 3$ \begin{array}{r | r} 2 & 160 \newline \hline 2 & 80 \newline \hline 2 & 40 \newline \hline 2 & 20 \newline \hline 2 & 10 \newline \hline 5 & 5 \newline \hline & 1 \end{array} $160=2^5 \times 5$ \begin{array}{r | r} 2 & 224 \newline \hline 2 & 112 \newline \hline 2 & 56 \newline \hline 2 & 28 \newline \hline 2 & 14 \newline \hline 7 & 7 \newline \hline & 1 \end{array} $224=2^5 \times 7$ $\therefore \text{HCF} = 2^5 = 2 \times 2\times 2\times 2\times 2 = 32$

Squares

The area of a square is given by the formula:
$\text{area}=\text{side}\times\text{side}$

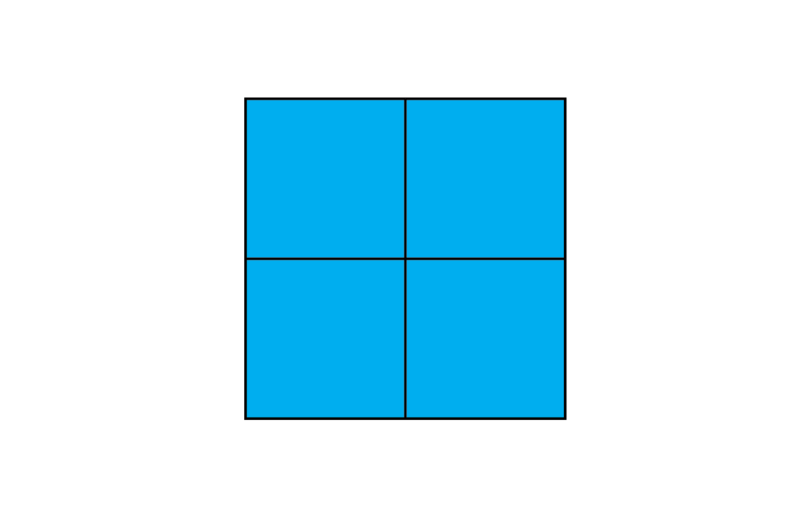
The area of the blue square is:
\begin{array}{r l} \text{area}&=\text{side}\times\text{side}\newline &=2\text{ blocks}\times 2\text{ blocks} \newline &=2^2\space\text{blocks} \newline &=4\space\text{blocks} \end{array}

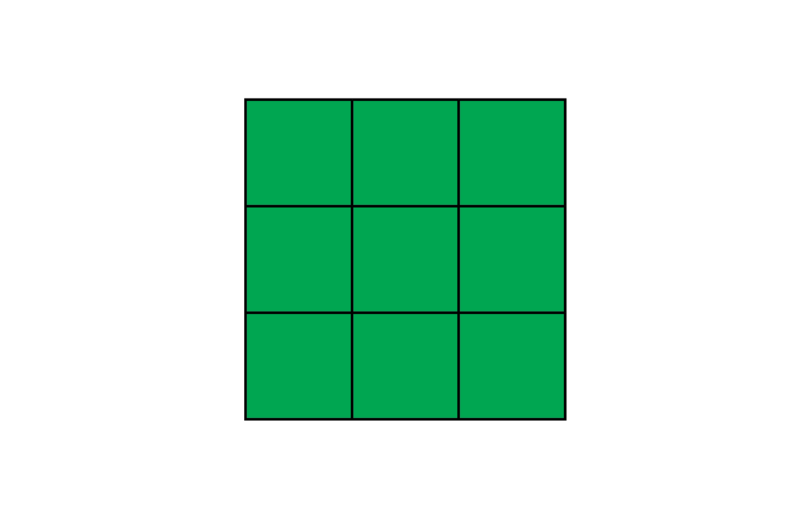
The area of the green square is:
\begin{array}{r l} \text{area}&=\text{side}\times\text{side}\newline &=3\text{ blocks}\times 3\text{ blocks} \newline &=3^2\space\text{blocks} \newline &=9\space\text{blocks} \end{array}

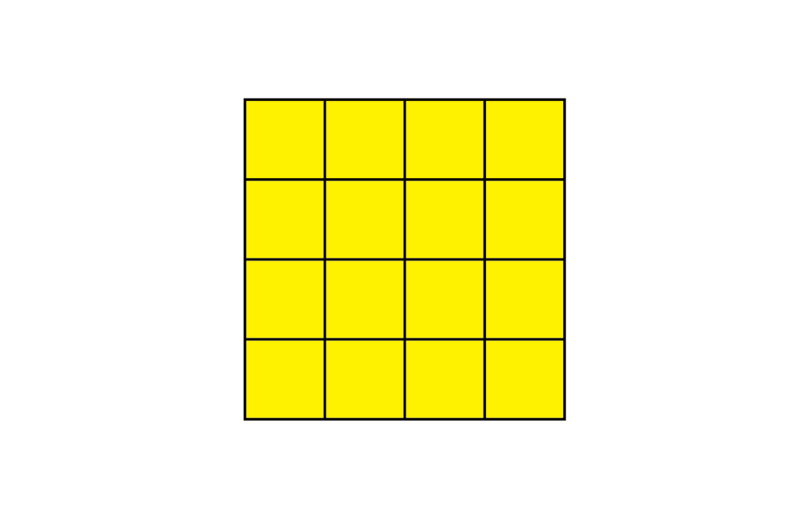
The area of the yellow square is:
\begin{array}{r l} \text{area}&=\text{side}\times\text{side}\newline &=4\text{ blocks}\times 4\text{ blocks} \newline &=4^2\space\text{blocks} \newline &=16\space\text{blocks} \end{array}

A perfect square is the answer we get when we multiply a whole number by itself. The first ten perfect squares are:

$\begin{array}{l} 1\times 1=1^2=1 \newline 2\times 2=2^2=4 \newline 3\times 3=3^2=9 \newline 4\times 4=4^2=16 \newline 5\times 5=5^2=25 \newline 6\times 6=6^2=36 \newline 7\times 7=7^2=49 \newline 8\times 8=8^2=64 \newline 9\times 9=9^2=81 \newline 10\times 10=10^2=100 \newline \end{array}$

A perfect square can always be expressed as the product of two identical factors. In index form, a perfect square may be expressed as a whole number to the power of 2, or the product of whole numbers to the power of 2.

perfect square A perfect square is the number that results from multiplying a whole number by itself.

While a perfect square is the result of a whole number multiplied by itself, we may find the square of any number, even if it is a fraction. For example, the square of 1.5 is $1.5\times 1.5=2.25$ . We say 1.5 squared is equal to 2.25.

Squares are shown with the power 2. For example, $7^2$ means "7 squared" or "the square of 7", which is 49.

square A square is the number that results from multiplying any number by itself.

Worked example 1.6: Finding perfect squares

Show that 80 is NOT a perfect square. Then find the smallest number by which 80 can be multiplied so that the answer is a perfect square.

Step 1: Express the number as a product of its prime factors.
\begin{array}{r | r} 2 & 80 \newline \hline 2 & 40 \newline \hline 2 & 20 \newline \hline 2 & 10 \newline \hline 5 & 5 \newline \hline & 1 \end{array} $80=2\times 2\times 2\times 2\times 5$
Step 2: Group similar prime factors in twos and write them in index form.
$80=2^2\times 2^2\times 5$
Step 3: Evaluate the powers of the grouped prime factors. If there are any numbers that are NOT to the power of 2, the number is NOT a perfect square.

In the prime factors of 80, 5 is to the power 1, which is NOT equal to 2. Therefore, 80 is NOT a perfect square.
Step 4: Work out by which prime number(s) we must multiply so that all the powers are equal to 2.
- In this case, we must multiply by 5, so that 5 is also to the power of 2: $2^2\times 2^2\times 5\times 5$
- The new grouped prime factors are: $2^2\times 2^2\times 5^2$
- We multiplied by an extra 5, so the new number is: $80\times 5=400$
- The factors are the numbers without the power of 2: $2 \times 2 \times 5 = 20$
- Check: $20 \times 20 = 400$

Exercise 1.6: Find perfect squares and calculate squares

Determine whether the following numbers are perfect squares or not. If not, find the smallest factor by which the number must be multiplied to get a perfect square.
1. 162
\begin{array}{r | r} 2 & 162 \newline \hline 3 & 81 \newline \hline 3 & 27 \newline \hline 3 & 9 \newline \hline 3 & 3 \newline \hline & 1 \end{array} $162=2\times 3\times 3\times 3\times 3=2\times 3^2\times 3^2$
The number 162 is NOT a perfect square. It must be multiplied by 2 to get a perfect square.
$\therefore 162\times 2=324$ is a perfect square.
1. 432
\begin{array}{r | r} 2 & 432 \newline \hline 2 & 216 \newline \hline 2 & 108 \newline \hline 2 & 54 \newline \hline 3 & 27 \newline \hline 3 & 9 \newline \hline 3 & 3 \newline \hline & 1 \end{array} $432=2\times 2\times 2\times 2\times 3\times 3\times 3=2^2\times 2^2\times 3^2\times 3$
The number 432 is NOT a perfect square. It must be multiplied by 3 to get a perfect square.
$\therefore 432\times 3=1,296$ is a perfect square.
1. 324
\begin{array}{r | r} 2 & 324 \newline \hline 2 & 162 \newline \hline 3 & 81 \newline \hline 3 & 27 \newline \hline 3 & 9 \newline \hline 3 & 3 \newline \hline & 1 \end{array} $324=2\times 2\times 3\times 3\times 3\times 3=2^2\times 3^2\times 3^2$
The number 324 is a perfect square.
Calculate the square of each of the following numbers.
1. 50
$50\times 50=2,500$
1. 0.8
$0.8\times 0.8=0.64$
1. $\frac{3}{5}$
$\frac{3}{5}\times \frac{3}{5}= \frac{9}{25}$

Square roots

A square root is the inverse of a square. You may think about it as the "opposite" of a square. The square root of a number is a factor of the number that, when multiplied by itself, gives the number.

square root A square root is the factor of a number that, when multiplied by itself, gives the number.

We use a special symbol to show square roots. For example, $\sqrt{49}$ means "the square root of 49". This is equal to the factor of 49 that, when multiplied by itself, gives 49. The factor is 7.

Do not confuse squares and square roots:
49 is the square of 7 and we write $7^2=49$
7 is the square root of 49 and we write $\sqrt{49}=7$

Worked example 1.7: Finding square roots of perfect squares

Find the square root of 784.

Step 1: Express the number as a product of its prime factors.
\begin{array}{r | r} 2 & 784 \newline \hline 2 & 392 \newline \hline 2 & 196 \newline \hline 2 & 98 \newline \hline 7 & 49 \newline \hline 7 & 7 \newline \hline & 1 \end{array} $784=2\times 2\times 2\times 2\times 7\times 7$
Step 2: Group similar prime factors in twos and write them in index form.
$784=2^2\times 2^2\times 7^2$
Step 3: The square root is equal to the product of the grouped prime factors without the powers of 2.
\begin{array}{l l} \sqrt{784}&=\sqrt{2^2\times 2^2\times 7^2}\newline &=2\times 2\times 7\newline &=28 \end{array}

Worked example 1.8: Finding square roots of fractions

Find the square root of $1\frac{104}{121}$ .

Step 1: Write the fraction as an improper fraction, in the form $\frac{\text{numerator}}{\text{denominator}}$ .
$1\frac{104}{121}=\frac{225}{121}$
Step 2: Determine the square root of the numerator and the square root of the denominator separately.
\begin{array}{l l} \sqrt{\frac{225}{121}}&=\frac{\sqrt{225}}{\sqrt{121}}\newline &=\frac{\sqrt{3^2\times 5^2}}{\sqrt{11^2}}\newline &=\frac{3\times 5}{11}\newline &=\frac{15}{11} \end{array}

Worked example 1.9: Finding square roots of decimal fractions

Find the square root of 0.000144.

Step 1: Write down the digits from the first non-zero digit to the last non-zero digit.

In this case, the digits are: 144
Step 2: Determine the square root of the number in Step 1.
\begin{array}{r | r} 2 & 144 \newline \hline 2 & 72 \newline \hline 2 & 36 \newline \hline 2 & 18 \newline \hline 3 & 9 \newline \hline 3 & 3 \newline \hline & 1 \end{array} $144=2^2\times 2^2\times 3^2$ \begin{array}{l l} \sqrt{144}&=\sqrt{2^2\times 2^2\times 3^2}\newline &=2\times 2\times 3\newline &=12 \end{array}
Step 3: Add zeros to your answer and insert a decimal point. You must have half the number of digits after the decimal point as there are in the original number.

The original number has 6 digits after the decimal point.
Therefore, the answer must have 3 digits after the decimal point: 0.012

Exercise 1.7: Calculate square roots

Calculate the square root of each perfect square.
1. 324
\begin{array}{r | r} 2 & 324 \newline \hline 2 & 162 \newline \hline 3 & 81 \newline \hline 3 & 27 \newline \hline 3 & 9 \newline \hline 3 & 3 \newline \hline & 1 \end{array} $324=2^2\times 3^2\times 3^2$ \begin{array}{l l} \sqrt{324}&=\sqrt{2^2\times 3^2\times 3^2}\newline &=2\times 3\times 3\newline &=18 \end{array}
1. 484
\begin{array}{r | r} 2 & 484 \newline \hline 2 & 242 \newline \hline 11 & 121 \newline \hline 11 & 11 \newline \hline & 1 \end{array} $484=2^2\times 11^2$ \begin{array}{l l} \sqrt{484}&=\sqrt{2^2\times 11^2}\newline &=2\times 11\newline &=22 \end{array}
1. 2,025
\begin{array}{r | r} 3 & 2,025 \newline \hline 3 & 675 \newline \hline 3 & 225 \newline \hline 3 & 75 \newline \hline 5 & 25 \newline \hline 5 & 5 \newline \hline & 1 \end{array} $2,025=3^2\times 3^2\times 5^2$ \begin{array}{l l} \sqrt{2,025}&=\sqrt{3^2\times 3^2\times 5^2}\newline &=3\times 3\times 5\newline &=45 \end{array}
Calculate the square root of each fraction.
1. $\frac{49}{900}$
$49=7^2$ \begin{array}{r | r} 2 & 900 \newline \hline 2 & 450 \newline \hline 3 & 225 \newline \hline 3 & 75 \newline \hline 5 & 25 \newline \hline 5 & 5 \newline \hline & 1 \end{array} $900=2^2\times 3^2\times 5^2$ \begin{array}{l l} \sqrt{\frac{49}{900}}&=\frac{\sqrt{49}}{\sqrt{900}}\newline &=\frac{\sqrt{7^2}}{\sqrt{2^2\times 3^2\times 5^2}}\newline &=\frac{7}{2\times 3\times 5}\newline &=\frac{7}{30} \end{array}
1. $\frac{400}{169}$
$169=13^2$ \begin{array}{r | r} 2 & 400 \newline \hline 2 & 200 \newline \hline 2 & 100 \newline \hline 2 & 50 \newline \hline 5 & 25 \newline \hline 5 & 5 \newline \hline & 1 \end{array} $400=2^2\times 2^2\times 5^2$ \begin{array}{l l} \sqrt{\frac{400}{169}}&=\frac{\sqrt{400}}{\sqrt{169}}\newline &=\frac{\sqrt{2^2\times 2^2\times 5^2}}{\sqrt{13^2}}\newline &=\frac{2\times 2\times 5}{13}\newline &=\frac{20}{13} \end{array}
1. $2\frac{34}{81}$
$2\frac{34}{81}= \frac{196}{81}$ $81=9^2$ \begin{array}{r | r} 2 & 196 \newline \hline 2 & 98 \newline \hline 7 & 49 \newline \hline 7 & 7 \newline \hline & 1 \end{array} $196=2^2\times 7^2$ \begin{array}{l l} \sqrt{\frac{196}{81}}&=\frac{\sqrt{196}}{\sqrt{81}}\newline &=\frac{\sqrt{2^2\times 7^2}}{\sqrt{9^2}}\newline &=\frac{2\times 7}{9}\newline &=\frac{14}{9} \end{array}
Calculate the square root of each decimal fraction.
1. 0.0121
\begin{array}{l l} \sqrt{121}&=\sqrt{11^2}\newline &=11 \end{array}
There must be 2 digits after the decimal point.
$\therefore \sqrt{0.0121}=0.11$
1. 0.0049
\begin{array}{l l} \sqrt{49}&=\sqrt{7^2}\newline &=7 \end{array}
There must be 2 digits after the decimal point.
$\therefore \sqrt{0.0049}=0.07$
1. 0.000256
\begin{array}{r | r} 2 & 256 \newline \hline 2 & 128 \newline \hline 2 & 64 \newline \hline 2 & 32 \newline \hline 2 & 16 \newline \hline 2 & 8 \newline \hline 2 & 4 \newline \hline 2 & 2 \newline & 1 \end{array} $256=2^2\times 2^2\times 2^2\times 2^2$ \begin{array}{l l} \sqrt{256}&=\sqrt{2^2\times 2^2\times 2^2\times 2^2}\newline &=2\times 2\times 2\times 2 \newline &=16 \end{array}
There must be 3 digits after the decimal point.
$\therefore \sqrt{0.0049}=0.016$

1.4 Practical applications

You may use your knowledge of multiples, factors, squares and square roots to solve problems. Here are some hints:

If you have to divide two or more amounts into smaller units, finding the HCF might solve the problem.
If you have to find a larger amount from smaller units, finding the LCM might solve the problem.
The square of a number is one of the number's multiples.
The square root of a number is one of the number's factors.

Exercise 1.8: Use factors, multiples, squares and square roots to solve problems

In 2019, Abeo is less than 50 years old. His age is a multiple of 5. In 2023, Abeo's age will be a multiple of 7, and he will still be younger than 50. What will Abeo's age be in 2023?

Multiples of 5 smaller than 50: $5;\space 10;\space 15;\space 20;\space 25;\space 30;\space 35; \space 40; \space 45; \space 50$
Multiples of 7 smaller than 50: $7;\space 14;\space 21;\space 28;\space 35;\space 42;\space 49$

In 2023, Abeo is 4 years older than in 2019. The number 49 (a multiple of 7) is 4 more than the number 45 (a multiple of 5). So Abeo will be 49 in 2023.

Note that the ages 10 and 14 are also 4 years apart, but someone of 14 years is not regarded as an adult.
The floor of a square room has a surface area of 6,561 cm $^{2}$ . The floor must be tiled with square tiles. The surface area of each tile is numerically the same as the side length of the room. Calculate how many tiles will be used.

\begin{array}{r | r} 3 & 6,561 \newline \hline 3 & 2,187 \newline \hline 3 & 729 \newline \hline 3 & 243 \newline \hline 3 & 81 \newline \hline 3 & 27 \newline \hline 3 & 9 \newline \hline 3 & 3 \newline \hline & 1 \end{array} $6,561=3^2\times 3^2\times 3^2\times 3^2$ \begin{array}{l l} \sqrt{6,561}&=\sqrt{3^2\times 3^2\times 3^2\times 3^2}\newline &=3\times 3\times 3\times 3\newline &=81 \end{array}
The side length of the room is 81 cm.
This means the area of one tile is 81 cm $^{2}$ .

$\therefore$ number of tiles = $\frac{6,561}{81}$ = 81 tiles
Two neighbouring schools both start their school day at 08h00. One school's periods are 36 minutes long and the other school's periods are 27 minutes long. They have first break at the same time. Determine the time at which first break starts.

Find the LCM of 36 and 27.

$36=2^2\times 3^2$
$27=3^3$
$\therefore\text{LCM}=2^2\times 3^3=2\times 2\times 3\times 3\times 3=108$

They have first break 108 minutes after 08h00. This is 1 hour and 48 minutes after 08h00.
Therefore, first break is at 09h48.
Two water tanks need to be filled. One tank has a capacity of 56 litres, and the other has a capacity of 84 litres. Water has to be carried over a distance to fill them. What volume container is needed to ensure that the journeys to the water source will be a minimum?

To keep the journeys to the water source at a minimum, the container that is used must have the maximum possible volume to fill each water tank an exact number of times.

Find the HCF of 56 and 84.

$56=2^3\times 7$
$84=2^2\times 3\times 7$
$\therefore\text{HCF}=2^2\times 7=28\text{ litres}$

The container must have a capacity of 28 litres.

1.5 Summary

Standard form is used to express large whole numbers and small fractions. A number is in standard form when it is written in the form , where 1\leqslant a<10 and is an integer.
- For whole numbers, the power $n$ is positive.
- For fractions, the power $n$ is negative.
A prime factor is a prime number that divides into a non-prime number without a remainder.
We can express any non-prime number as a product of its prime factors.
Index form is a way of writing as a superscript how many times a number is multiplied by itself.
The lowest common multiple (LCM) is the smallest whole number into which two or more numbers divide without a remainder.
The highest common factor (HCF) is the largest whole number that divides into two or more numbers without a remainder.
When we express two or more numbers as prime factors in index form:
- the HCF is the product of the common prime factors to the lowest index
- the LCM is the product of all the prime factors to the highest index.
A perfect square is the number that results from multiplying a whole number by itself.
The square of a number is the number that results from multiplying any number by itself. It is one of the multiples of the number.
The square root of a number is the factor of the number that, when multiplied by itself, gives the number. It is one of the factors of the number.

Table of Contents

Chapter 2: Fractions

Test yourself now

Chapter 1: Numbers big and small

1.1 Standard form

Expressing whole numbers in standard form

Worked example 1.1: Writing large numbers in standard form

Exercise 1.1: Convert between ordinary whole numbers and standard form

Expressing decimal fractions in standard form

Worked example 1.2: Writing decimal fractions in standard form

Exercise 1.2: Convert between ordinary decimal fractions and standard form

1.2 Prime factorisation

Worked example 1.3: Revising prime factors

Exercise 1.3: Express numbers as products of prime factors in index form

1.3 Applications of prime factorisation

Lowest common multiple (LCM)

Worked example 1.4: Revising LCM

Exercise 1.4: Determine LCM

Highest common factor (HCF)

Worked example 1.5: Revising HCF

Exercise 1.5: Determine HCF

Squares

Worked example 1.6: Finding perfect squares

Exercise 1.6: Find perfect squares and calculate squares

Square roots

Worked example 1.7: Finding square roots of perfect squares

Worked example 1.8: Finding square roots of fractions

Worked example 1.9: Finding square roots of decimal fractions

Exercise 1.7: Calculate square roots

1.4 Practical applications

Exercise 1.8: Use factors, multiples, squares and square roots to solve problems

1.5 Summary